BOLYAI'S LAST MAJOR WORK
BOLYAI, FARKAS.
A’ Marosvasarhelyt 1829-be nyomtatott Arithemetika Elejének részint röviditett, reszint bovitett, általán jobbitott, ‘s tisztáltabb kiadása.
Marosvásárhely, Kali Simon, 1843.
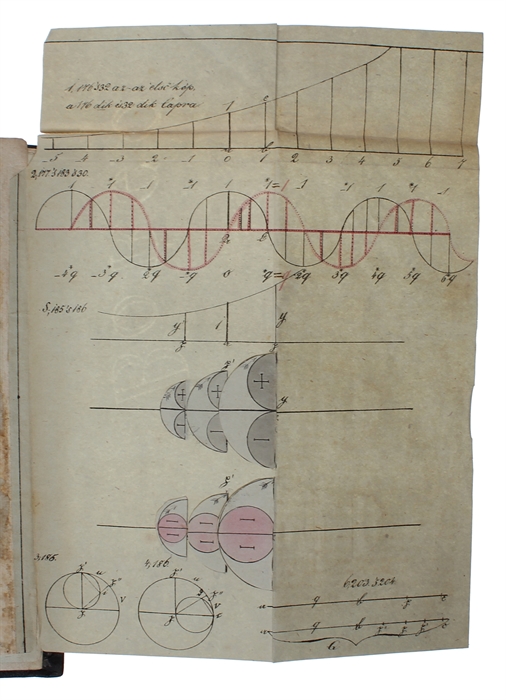
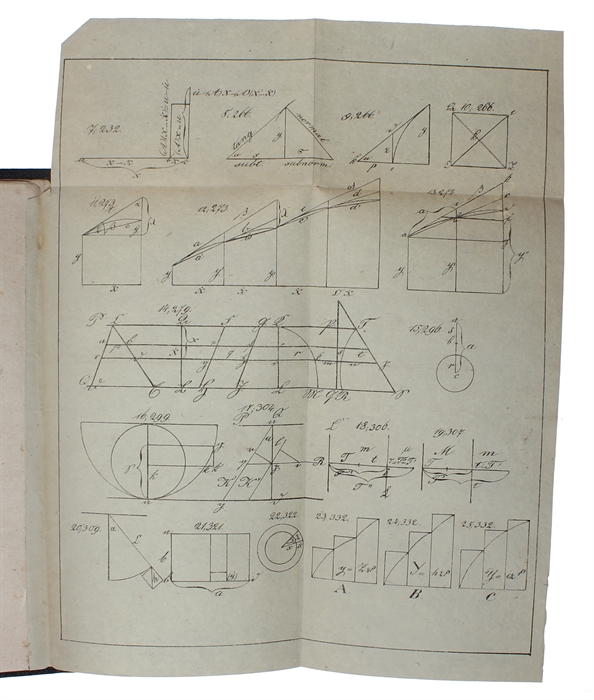
8vo. In a simple contemporary half calf with gilt ornamentation to spine forming five compartments. Later paper title-label with gilt lettering pasted on to spine, partly detached in right margin. Light wear to extremities. Stamp to front free end-paper. First leaves evenly lightly browned. An overall fine and clean copy. XLIV, 386 pp. + 2 folded plates, one with 12 folding flaps with partial grey colouring.
The rare first edition of Bolyai’s important work on the foundations of mathematics, being his last major work. It is in part based on his ‘Az arithmethica eleje’ (1830), in many aspects a rudimentary and introductory work, and the second volume of his magnum opus ‘Tentamen juventutem studiosam elementa matheseos purae’ (1832-33) – but here, for the first time, expanded and fully expounded. As with Bolyai’s other works, it was unappreciated by his contemporaries: “He can be taken as a precursor of Gottlob Frege, Pasch, and Georg Cantor; but, as with many pioneers, he did not enjoy the credit that accrued to those that followed him” (DSB). His work was considered mathematically incomprehensible by his colleagues and only his students and his son, János Bolyai, understood and appreciated it. Probably because of lack of interest from Bolyai’s contemporaries, all of his works are now rare, the present work being no exception. It has appeared only once at auction the past 30 years. The Tentamen itself, the fundamental ideas of which may date back to Bolyai’s Göttingen days, is an attempt at a rigorous and systematic foundation of geomentry (Volume I) and of arithmetic, algebra, and analysis (Volume II). The huge work shows the critical sprit of a man who recognized, as did few of his contemporaries, many weaknesses in the mathematics of his day, but was not able to reach a fully satisfactory solution of them." (DSB) Neverthless, when it is remembered that Bolyai worked in almost total isolation, his works are a most remarkable witness to the sharpness of his mind and to his perseverance. Not in Sommerville
In 1796, Farkas Bolyai (1775-1856) traveled to Germany, first to Jena and then to Göttingen, where he studied until 1799. It was at this time that Bolyai began his lifelong friendship with Carl Friedrich Gauss, also a student at the University Göttingen, who was already intensely engaged in mathematical research. Bolyai’s interest in the foundations of geometry dates from this period, especially in the so-called Euclidean or parallel axiom, to which Kastner and Seyffer, as well as Gauss were devoting their attention. Bolyai maintained a correspondence with Gauss that, with interruptions, lasted all their lives.
Bolyai accepted the position of professor of mathematics, physics, and chemistry at the Evangelical-Reformed College at Marosvásárhely in 1804, where he taught until his retirement in 1853. Meanwhile, he continued his research, concentrating on the theory of parallels. He sent a manuscript on this subject, Theoria parallelarum, with an attempt to prove the Euclidean axiom, to Gauss in 1804. The reasoning, however, satisfied neither Gauss nor himself, and Bolyai continued to work on it and on the foundations of mathematics in general.
“In 1829 Bolyai finished his principal work, but because of technical and financial problems it was not published until 1832–1833. It appeared in two volumes, with the title Tentamen juventutem studiosam in elementa matheseos purae, elementaris ac sublimioris, method intuitiva, evidentiaque huic propria, introducendi, cum appendice triplici (“An Attempt to Introduce Studious Youth Into the Elements of Pure Mathematics, by an Intuitive Method and Appropriate Evidence, With a Threefold Appendix”). While writing the Tentamen, Bolyai had his first difficulties with his son János. In spite of warnings from his father to avoid any preoccupation with Euclid’s axiom, János not only insisted on studying the theory of parallels, but also developed an entirely unorthodox system of geomentry based on the rejection of the parallel axiom, something with which his father could not agree. However, despite misgivings, Bolyai added his son’s paper to the first volume and thus, unwittingly, gave it immortality. In 1834, a Hungarian version of Volume I was published.
Order-nr.: 60233