WESSEL, CASPAR. - THE FIRST SYSTEMATICAL THREATMENT OF COMPLEX NUMBERS.

Om Directionens analytiske Betegning, et forsøg, anvendt fornemmelig til plane og sphæriske Polygoners Opløsning. (Essai sur la représentation analytique de la direction, avec des applications, en particular à la détermination des polygones plans et des polygones sphériques).

Kiøbenhavn, Johan Rudolph Thiele, (1797) 1799.

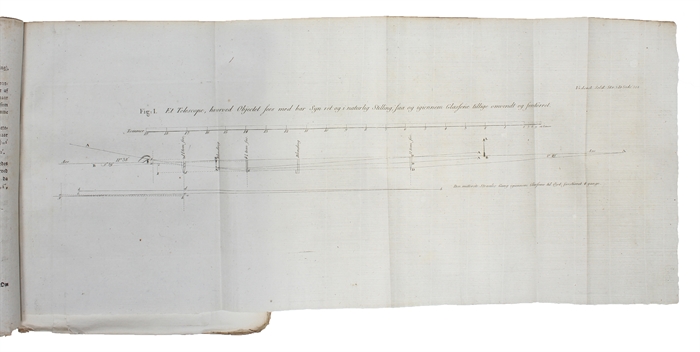
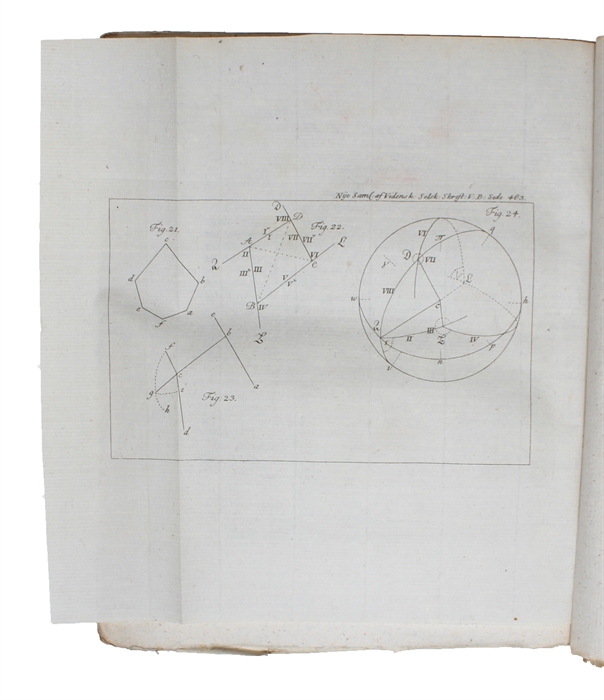
4to. Uncut and unopened in original blue boards. Published in: "Nye Samling af det Kongelige Danske Videnskabernes Selskabs Skrifter." Vol. V. Wessel's paper: pp.469-518 and 3 folded engraved plates (the last plate inserted at p. 463). The whole volume V offered in its original binding. Engraved titlevignette. XII,670 pp., 15 engraved plates. 4 leaves with upper right corners gone, not affecting Wessel's paper.

First edition of this important first systematical treatment of the theory of complex numbers and at the same time, the first work to add vectors in three-dimensional space.

"Wessel’s fame as a mathematician is based entirely on one paper, written in Danish and published in the Mémoires of the Royal Danish Academy, that established his priority in publication of the geometric representation of complex numbers. John Wallis had given a geometric representation of the complex roots of quadratic equations in 1685; Gauss had had the idea as early as 1799 but did not explicitly publish it until 1831. Robert Argand’s independent publication in 1806 must be credited as the source of this concept in modern mathematics because Wessel’s work remained essentially unknown until 1895, when its significance was pointed out by Christian Juel.

The title of Wessel’s treatise calls it an "attempt" to give an analytic representation of both distance and direction that could be used to solve plane and spherical polygons. The connection of this goal with Wessel’s work as a surveyor and cartographer is obvious. The statement of the problem also suggests that Wessel should be credited with an early formulation of vector addition. In fact, Michael J. Crowe, in A History of Vector Analysis (University of Notre Dame Press, 1967), defines the first period in that history as that of a search for hypercomplex numbers to be used in space analysis and dates it from the time of Wessel, whom he calls the first to add vectors in three-dimensional space." (DSB).

Order-nr.: 58364